Longest Consecutive Subsequence: Codes with Visualization
Sasank is a Technical Content Writer with a strong background in competitive programming. He has solved nearly 1500 problems on LeetCode and achieved top rankings in LeetCode Weekly Contests, including global ranks of 171, 196, 206, 308, 352, and more. His expertise in algorithms and problem-solving is also reflected in his active participation on platforms like Codeforces. Sasank enjoys sharing his technical knowledge and helping others learn by creating clear and accessible content.
Problem Statement
Given an unsorted array of integers, find the length of the longest consecutive elements sequence.
For example, if the input array is [100, 4, 200, 1, 3, 2], the longest consecutive subsequence is [1, 2, 3, 4], so the output should be 4.
Important note: The algorithm should run in O(n) time complexity.
Brute Force Approach
Explanation
The simplest way to approach this problem is to:
- Sort the array
- Iterate through it, counting consecutive elements
While this isn't the most efficient solution, it's a good starting point to understand the problem.
After sorting, we can scan through the array and keep track of the current streak of consecutive numbers. Whenever we find a gap (current number is not one more than the previous), we reset our counter. We'll keep track of the maximum streak length we've seen so far.
Time Complexity: O(n log n) due to the sorting operation
Space Complexity: O(1) if we sort in place, or O(n) if we create a new sorted array
Python Code
def longest_consecutive_brute(nums):
if not nums:
return 0
# Sort the array
nums.sort()
longest_streak = 1
current_streak = 1
# Iterate through the sorted array
for i in range(1, len(nums)):
# If current element is different from previous (to handle duplicates)
if nums[i] != nums[i-1]:
# If current element is consecutive to previous
if nums[i] == nums[i-1] + 1:
current_streak += 1
else:
current_streak = 1
longest_streak = max(longest_streak, current_streak)
return longest_streak
C++ Code
#include <vector>
#include <algorithm>
int longestConsecutiveBrute(std::vector<int>& nums) {
if (nums.empty()) {
return 0;
}
std::sort(nums.begin(), nums.end());
int longest_streak = 1;
int current_streak = 1;
for (int i = 1; i < nums.size(); i++) {
if (nums[i] != nums[i-1]) {
if (nums[i] == nums[i-1] + 1) {
current_streak++;
} else {
current_streak = 1;
}
}
longest_streak = std::max(longest_streak, current_streak);
}
return longest_streak;
}
Java Code
import java.util.Arrays;
public class Solution {
public int longestConsecutiveBrute(int[] nums) {
if (nums.length == 0) {
return 0;
}
Arrays.sort(nums);
int longestStreak = 1;
int currentStreak = 1;
for (int i = 1; i < nums.length; i++) {
if (nums[i] != nums[i-1]) {
if (nums[i] == nums[i-1] + 1) {
currentStreak++;
} else {
currentStreak = 1;
}
}
longestStreak = Math.max(longestStreak, currentStreak);
}
return longestStreak;
}
}
Optimized Approach
Explanation
The brute force approach works, but it doesn't meet the O(n) time complexity requirement because of the sorting operation. We can do better using a HashSet.
The key insight is that we can use a set to quickly check if a number exists in our collection. Here's the optimized approach:
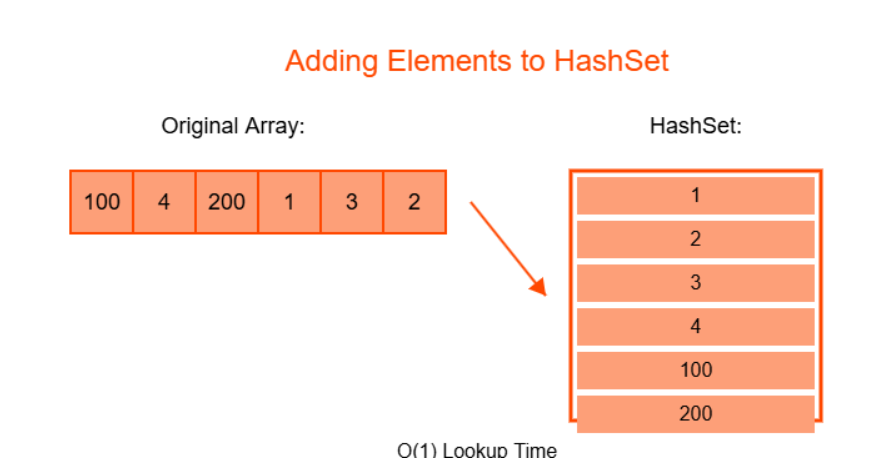
- Insert all elements of the array into a HashSet
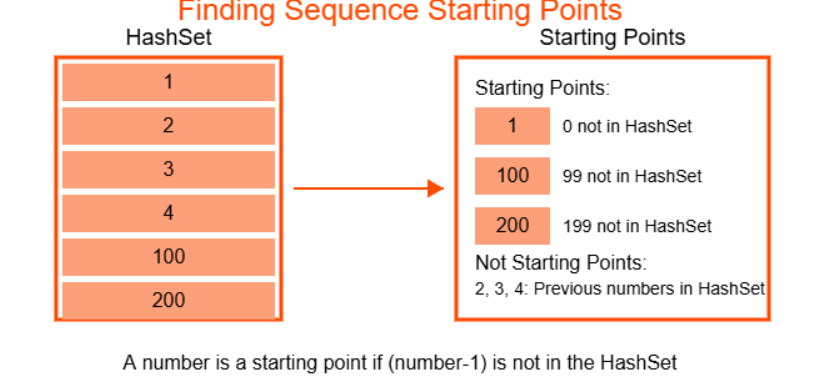
- For each number in the array, check if it's the start of a sequence (i.e., if num-1 is not in the set)
- If it is a starting point, count how many consecutive elements follow it
- Keep track of the maximum sequence length
This approach works in O(n) time because:
- Building the HashSet takes O(n) time
- We do at most 2n lookups in the HashSet (checking for each number and its consecutive numbers)
- HashSet lookups are O(1) on average
Time Complexity: O(n)
Space Complexity: O(n) for the HashSet
Let's consider the array [100, 4, 200, 1, 3, 2].
num = 1
- 0 not in num_set → it's a sequence start
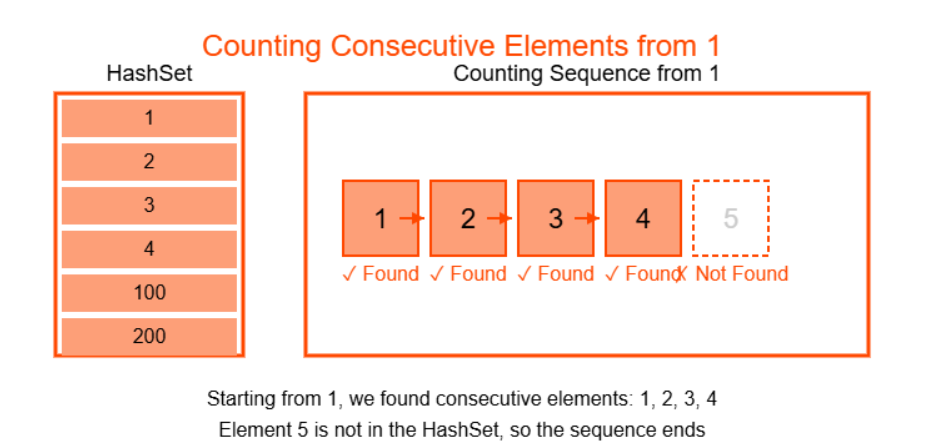
- current_num = 1, current_streak = 1
- 2 in num_set → current_num = 2, streak = 2
- 3 in num_set → current_num = 3, streak = 3
- 4 in num_set → current_num = 4, streak = 4
- 5 not in num_set → end sequence
longest_streak = max(0, 4) = 4
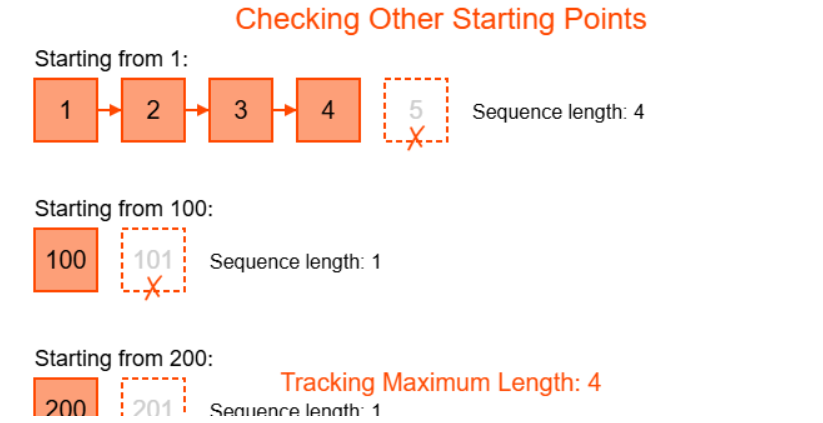
num = 2, 3, 4
- All of these have (num - 1) in the set → skip (not start of a new sequence)
num = 100
- 99 not in num_set → start of new sequence
- 101 not in num_set → streak = 1
longest_streak = max(4, 1) = 4 (unchanged)
num = 200
- 199 not in num_set → start of new sequence
- 201 not in num_set → streak = 1
longest_streak = max(4, 1) = 4 (unchanged)
<visualization-box>
Python Code
def longest_consecutive(nums):
if not nums:
return 0
# Create a set of all numbers for O(1) lookups
num_set = set(nums)
longest_streak = 0
for num in num_set:
# Check if it's the start of a sequence
if num - 1 not in num_set:
current_num = num
current_streak = 1
# Count consecutive numbers
while current_num + 1 in num_set:
current_num += 1
current_streak += 1
longest_streak = max(longest_streak, current_streak)
return longest_streak
C++ Code
#include <vector>
#include <unordered_set>
#include <algorithm>
int longestConsecutive(std::vector<int>& nums) {
if (nums.empty()) return 0;
std::unordered_set<int> num_set(nums.begin(), nums.end());
int longest_streak = 0;
for (int num : num_set) {
if (num_set.find(num - 1) == num_set.end()) {
int current_num = num;
int current_streak = 1;
while (num_set.find(current_num + 1) != num_set.end()) {
current_num++;
current_streak++;
}
longest_streak = std::max(longest_streak, current_streak);
}
}
return longest_streak;
}
Java Code
import java.util.HashSet;
import java.util.Set;
public class Solution {
public int longestConsecutive(int[] nums) {
if (nums.length == 0) return 0;
Set<Integer> numSet = new HashSet<>();
for (int num : nums) {
numSet.add(num);
}
int longestStreak = 0;
for (int num : numSet) {
if (!numSet.contains(num - 1)) {
int currentNum = num;
int currentStreak = 1;
while (numSet.contains(currentNum + 1)) {
currentNum++;
currentStreak++;
}
longestStreak = Math.max(longestStreak, currentStreak);
}
}
return longestStreak;
}
}
Conclusion
In this article, we learned how to find the longest consecutive subsequence in an array. We started with a brute force approach using sorting, which gave us an O(n log n) solution. Then, we optimized it to an O(n) solution using a HashSet, meeting the problem's requirement.
The key takeaway from this problem is how using the right data structure can dramatically improve the efficiency of our algorithm. By using a HashSet to enable O(1) lookups, we transformed what would have been an O(n²) or O(n log n) problem into a linear time solution.
